Q. A photon traveling in the positive x-direction collides with a stationary free electron. The incident photon has a wavelength of 0.0310 nm. Post-collision, the electron moves at an angle 𝛼 below the positive x-axis, and the photon deflects at an angle 𝜃 = 66.3° above the positive x-axis. A) Calculate the angle 𝛼 (in degrees). B) Compute the velocity of the electron (in m/s). Solution:
Previously we discussed motion in a straight line. Motion in straight line belongs to one dimension. Thus, Motion in plane defined in two dimensions. If an object is free to move in a plane, its position can be located with two co-ordinates. Let's consider an object is situated in x-y plane. It moves along the following path-
During the path object moves along X direction as well as Y direction. In time interval t to t+t1 (here t1 is very small time interval) object displaced x1 and y1 in X and Y direction respectively. Displacement is in both X and Y direction, so there will be different velocity and acceleration in both directions.
If we take ux ,vx ,ax as initial velocity ,final velocity and acceleration in X direction and uy ,vy ,ay as initial velocity ,final velocity and acceleration in Y direction. Consider both the accelerations constant. In time t object have 'x' and 'y' displacements along X-axes and Y-axes respectively. Then equations of motion for both directions can be written as-
Most popular examples of plane motion are projectile motion and circular motion.
Projectile Motion:
When an object is thrown obliquely near the earth’s surface, it moves along a curved path under constant acceleration (gravitational acceleration, near the surface we take it as constant, g= 9.8 m/s²) that is directed towards the center of the earth. Such a path is known as projectile and motion of object is called as projectile motion. Most common example of projectile motion in football match we see ball travels in projectile path. See in the clip.
Below the projectile motion of an object is shown-
An object is projected with a initial velocity u at an angle θ from horizontal. Since object has both horizontal as well as vertical component of velocity so, it's displacement will be in both direction.
In y-direction particle is moving under constant gravitational acceleration g towards the center of the earth. In y-direction initial velocity uy = u sinθ, final velocity would be zero. So, maximum height H can be derived as-
Time of flight, T is total time taken during the projectile path. We know that object has same magnitude of acceleration in vertically upward and vertically downward. Consider 't' is time to reach at maximum height of projectile. So time of flight is equal to 2t. In vertically upward direction-
Object has no acceleration in x-direction. So it's velocity will be same in x-direction during the motion. Maximum distance covered by the object in x-direction is called as horizontal range, R. Initial velocity in x-direction ux = u cosθ. It will be unchanged during the whole path.
Relation between R (horizontal range) and H (maximum height):
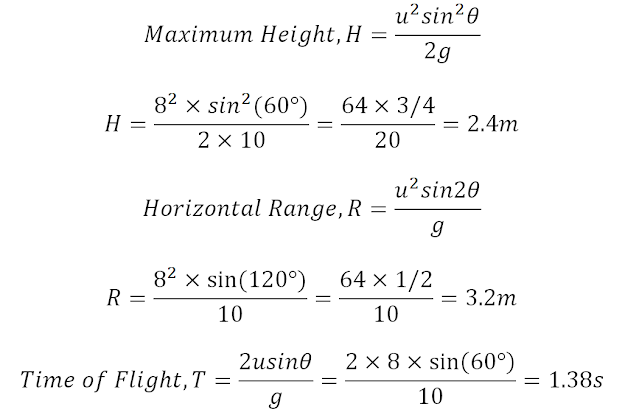
Example: A ball is thrown from ground with a speed of 8 m/s at an angle of 60 degree from horizontal. Find it's maximum height, horizontal range and time of flight ? (take g = 10 m/s²)
Solution:
Very nyc concept
ReplyDelete